[tutorials & resource material arranged by topic Class Table [next] [last]

MIDDLE GROUND - Stanine
-- Statistical Standard Nine Normal Distribution


A Standard Normal Distribution
With Nine Specific Intervals
 
      There exists a normal distribution with a mean of 0 and a standard deviation of 1. It is called the standard normal distribution.
 
      When the center interval is within a quarter of a standard deviation of the mean, and each of the other intervals are a half standard deviation wide (exclusive of the tails), the distribution has been marked in stanines -- the standard nine intervals.
    It is often used to:
  • compare two or more distributions of data, particularly test scores.
  • estimate or to compute probabilities of events involving normal distributions,
  • facilitate using words rather than numbers in presenting statistical data.
[Click on graphic to go to a printable copy.]
      The standard normal distribution and scale may be thought of as a tool to scale up or down another normal distribution.
 
      The standard normal distribution is a tool to translate a normal distribution into numbers which may be used to learn more information about the set of data than was originally known.
 
      The stanine interevals are a tool to put descriptive words in place of numbers and also to create enough intervals so that conversation about and comparison of two or more sets of scores is meaningful.
 
      It is still a standard normal distribution, so the same notations and variables hold: a standard normal scores (z), the normal distribution mean (either 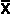or µ), the normal distribution standard deviation, (either s or 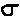), and the normal distribution scores (x), are used and
            and     .
 
      The difference is now words and less accuracy are used. For example:
 
      "Terry tested considerably above average."
 
      That means his/her score was in the 7th stanine.
 
 
Between, Below, Above, and Not Between

      It may be clear to the reader that providing more intervals with probabilities also means the problems may increase slightly in complexity, but, still in general are confined to 3 or 4 general types. The fourth being "not in the interval between score a and score b."
      These problems may be completed using the arithmetic aides of the last page -- to compute x or z and to label the distribution axis -- and also the statistics papers linked there, or the stanine w/percent papers here.
     
Questions with answers and work.
      Given normally distributed scores with a mean of 0 and standard deviation of 1.
 
a. What percent of scores are between -.75 and .25?
b. What percent of scores are greater than 1.25?
 
 
Questions with Answer Page
 
1. Explain the word stanine.
 
2. Given the standard normal distribution, compute the probabilites.
a. p(-1.75 < z < -.25)      
b. p(z is not in the interval -1.75 < z < -.25)
c. p(z < -1.25)
 
3. Given a normal distribution with a mean of 65 and a standard deviation of 10,
a. estimate p(52.5 < x < 62.5)      
b. 1 - p(x < 72.5
c. p(x > 72.5)
 
4. Each year students are tested and parents wish to understand the scores. This year's math grades were normally distributed with a mean of 65 and a standard deviation of 10. Use stanines to explain to the parents how well their chidren did if the scores for 4 children were 98, 23, 66, and 80.
 
  answers [next] [last]
[MC,i. Home] [Table] [Words] Classes [this semester's schedule w/links] [Good Stuff -- free & valuable resources] [next] [last]
© 2005, Agnes Azzolino
www.mathnstuff.com/math/spoken/here/2class/90/stanine.htm