MIDDLE GROUND - Some Features of A Normal Distribution

 

A Normal Frequency Distribution         The last page said, "the word normal is a very powerful adjective when used to describe a frequency distribution or when used to describe the data of a sample or population."   This page explains the things one knows and is guaranteed as soon as one learns a set of data is normally distributed.         If you have not done so already, please review/read the last page on the meaning of a statistic.

 

 

The Data Is Centered About the Mean-Mode-Median         All normal distributions "look like" the one above. The mean-mode-median is in the center. It is the mean because it is the ARITHMETIC average of all the scores. It is the mode because of all the scores the mean score happens MOST often. It is the median because when the scores are displayed from lowest to highest, the mean is the MIDDLE score, the median. The EXPECTED value is the mean. The frequency curve is bell shaped. The bell shape has perfect bilateral symmetry - the left balances exactly with the right. The score at -2 is balanced by a score at +2 and the frequencies from 0 to +2 and from 0 to -2 are equal. The area under the curve from 0 to +2 is exactly the same as the area under the curve from 0 to -2. Fifty percent of the scores are above the mean and 50% are below the mean. The probability a score is above the mean is 50% and the probability a score is below the mean is 50%. Most of the scores are in the middle, about the mean, and few are in the tails, at the extremes. The area under the curve is equal to 1. The probability of an event that does not happen is 0. The sum of the probabilities of all events is 1. The standard deviation tells one how the scores are spread out and therefore the fatness or skinniness of the bell. Because "the shape" of one normal distribution is "the shape" of all others, the spread of the area of one normal distribution "is the same" as all others and the standard deviation is the scale. The frequencies for the set of scores with a normal distribution are stated by a function which includes as controlling features both the mean, µ, and the standard deviation, 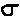, of the set of scores. That function is:

 

 

Probability and A Normal Distribution         Below is the frequency distribution of statistically normal data.         The number line is marked in terms of the mean and the standard deviation.         Since normally distributed data is spread in terms of the mean and standard deviation, the percent of scores, within the same region, remains the same from normal distribution to normal distribution.

 

 

Probabilities and Areas Under the Curve Are Known         The areas, the probabilities, may be grouped and added as desired.

 

 

Use Information on This Page to Answer These Questions   1. Complete: The mode of a normal distributions is ___.   2. Complete: _____ percent of a scores in a normal distribution are above the mean.   3. In the figure above, the symbols p(-2 < z < 2) is read, "the probability a standard normal score is between -2 and 2," or, "the probability a standard normal score is within 2 standard deviations of the mean." State this probability as a number.   4. For a standard normal distribution, state: a. p(z > 0)       b. p(z < 0) c. p(z = 0) d. p(z > 2) e. 1 - p(z > 2) f. p(z < 2) g. p(-3 < z < 3) h. p(-2 < z < -1) i. p(z < -1)   5. A normal distribution has a mean of 10 and a standard deviation of 2. What's the probability a. a score is within 1 standard deviation of the mean? b. a score is between 10 and 12?

 

  (answers)    

© 2005, Agnes Azzolino www.mathnstuff.com/math/spoken/here/2class/90/normal.htm