The Languages of the Math Classroom

© '96 & '98, Agnes Azzolino

The Semi or Pseudo Expression or Equation

Questions 5 and 6 below are from the Warmup. Please complete them now if you and your partner have not done so already.

Please solve the following problems on paper. Show work for possible partial credit.

Then, discuss your answer and work with your partner.

5.	Compute the change from a fifty dollar bill for the purchase of 3 notebooks at $2.90 each and 2 CDs at $14.50 each.
6.	Three consecutive integers are involved. The sum of the first, triple the middle, and four times the largest is 131. Find the integers.

My work on each problem is displayed below. Your work probably looks similar but probably does not include the top line of work - the semi or pseudo equation or expression.

	   5. Compute the change from a fifty dollar bill for 
	the purchase of 3 notebooks at $2.90 each and 2 CDs 
	at $14.50 each.

		     50 - 3(notebooks) - 2(CDS)
		     50 - 3(2.90) - 2(14.50) 
		     $32.60 is the change

	   6. Three consecutive integers are involved.  The 
	sum of the first, triple the middle, and four times 
	the largest is 131.  Find the integers.

		 (first) + 3(second) + 4(third)	= 131
		 (x)     + 3(x + 1) + 4(x + 2)	= 131
				      8x + 11		= 131
				      8x			= 120
				      x			= 15
			the numbers are 15, 16, 17

My assumption is that we all got the correct answer.

Now, who earned the most credit for displaying the work?

DTWYP, Discuss this with your partner.

Semi/Pseudo Expression/Equation

The semi/pseudo code looks like this and is "half symbols, half words."

		     50 - 3(notebooks) - 2(CDS)

		 (first) + 3(second) + 4(third)	= 131

I use the semi/pseudo code for the following reasons:

To present a unified and complete representation of the problem.
To facilitate, through this intermediate step, the transition from words to mathematical symbolic notation.
To foreshadow computation on graphing or symbol manipulating calculators.
Because it helps my students "decode" a word problem!

Here are the rules:

Use words (including nicknames) and arithmetic to express an idea.

Perhaps encircle the words with parentheses for clarity.
Use no variable numbers. Use only constant numbers.
Use order of operations.

Your turn to try it!

Just write the semi- or pseudo- code and then the equation.

Please solve the following problems on paper. Show work for possible partial credit.

7.	The product of two consecutive even integers equals four more than double the sum of the even integers. Find the integers.
8.	Compute the area.

Here's my work.

	7.
		(first)(second)	= 2(sum) + 4
		(first)(second)	= 2(first + second) + 4
		(x)(x + 2) 		= 2(x + x + 2) + 4
	         x² + 2x		= 4x + 4 + 4
           x² - 2x - 8 		= 0 
          (x - 4)(x + 2) 	= 0
	         x - 4 	= 0	or	  x + 2	= 0
        	      x	= 4    	     x 	= -2

	 The numbers are
		 4 and 6  	or	-2 and 0.

How'd it go?

Please discuss this with your partner.

The semi or pseudo equation represents the type of work I wish to see on every test in an arithmetic, general math, pre-algebra, algebra, statistics, or calc course. It lets the reader know exactly what the writer understands the desired procedure to be. It explains the meaning of the algebraic expression or equation below it, and, represents it in a form consistent with the standard presentation of algebraic computation. It presents a master plan for the solution of the problem and though coded in words and arithmetic or algebraic symbols, follows order of operations, the grammar of mathematics.

The semi/pseudo equation/expression is a powerful language with which to communicate! It is the written language which bridges the mother tongue and formal mathematics. If you've never used

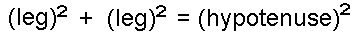
in an elementary math class you may be surprised at how well the semiequation works.

Used in an arithmetic class, it is a precursor to algebra. Use in any math class, it is a precursor to work with a graphing calculator, is identical to the types of pseudocoding used in modern computer languages, and recalls the traditions of early algebra.

Without the semi/pseudo code or a complete expression of the relation between all important values in the computation of the answer, the work is reminiscent of papers appropriate in an elementary school 30 years ago. The stage has not been set for algebraic expression. The work is not ready for calculator use. The semi/pseudo code exemplifies work of a student prepared in the present and ready for the present and future.

The semi/pseudo expression/equation are not products of the 20th century, these are the precursor of 20th century algebra - the transitional stages from the written word to the written symbol. Clearly a blend of word and symbols has a prestigious history. The Rhind Mathematical Papyrus [NCTM, 1979] -as in math of Ancient Egypt -- states in problem 24:

A quantity, 1/7 of it added to it, becomes it : 19.

The + symbol for addition was not even invented by Johann Widman until 1489. Robert Recorde did not invent the = sign till 1557. "Before these dates ... words were used instead of symbols" [Usiskin, 1976].

Only recently has the education community recognized the power of manipulatives in teaching mathematics. Yet 5,000 years ago, the probable precursor to clay tablet markings and written symbols, the token, a manipulative, was used [Peterson, 1988]. Now it is time to revive another teaching strategy, the semi-equation.

Aren't we now ready to take a step back to the future and accept or at least experiment with teaching math with manipulatives, semi-expressions and semi-equations? Don't dwell in the past unless you use it to build the future. The future lies with manipulatives, with the calculator, and with semi-equations.

I'm interested in your reaction. I'd love to hear from you. You might use my email at asquared@mathnstuff.com or the form below.

References

[Table of Contents] [Top of the Page] [Last Page] [Next Page]
[Verbal] [Written] [Pictorial] [Concrete]