-
Questions with Answer Page
-
- 1. State the area under the standard normal curve
- between z-scores of 0 and 1.42.
-
- 2. Given the standard normal distribution, compute
- p(z is within 1.42 standard deviations of the mean),
- p(-1.42 < z < 1.42).
-
- 3. Given the standard normal distribution, find the z-score such that
- p(z is within __ standard deviations of the mean) = 95%.
-
- 4. Find, to two decimal places accuracy, the boundaries
- in the standard normal distribution, such that
- p(z is within __
standard deviations of the mean) = 74.98%.
-
- 5. Using the z-scores in the above table, state the lowest z-score
- which is in the top 90% of all scores.
-
- 6. Using the z-scores in the above table, state the lowest z-score
- which is in the top 85% of all scores.
-
- 7. Compute:
- p(-2.2 < z < -2.35).
-
- 8. Compute,
- given a normal distribution,
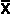 = 3 and s = 0.4, = 3 and s = 0.4,
- p(2 < x < 4).
|

![[next]](http://www.mathnstuff.com/math/spoken/here/1gif/next.gif)
![[last]](http://www.mathnstuff.com/math/spoken/here/1gif/last.gif)



![[next]](http://www.mathnstuff.com/math/spoken/here/1gif/next.gif)
![[last]](http://www.mathnstuff.com/math/spoken/here/1gif/last.gif)

![[MC,i. Home]](http://www.mathnstuff.com/math/spoken/here/1gif/mcihome.gif)
![[Table]](http://www.mathnstuff.com/math/spoken/here/1gif/table.gif)
![[Words]](http://www.mathnstuff.com/math/spoken/here/1gif/words.gif)

![[this semester's schedule w/links]](http://www.mathnstuff.com/gif/semestr.gif)
![[Good Stuff -- free & valuable resources]](http://www.mathnstuff.com/gif/goods.gif)
![[next]](http://www.mathnstuff.com/math/spoken/here/1gif/next.gif)
![[last]](http://www.mathnstuff.com/math/spoken/here/1gif/last.gif)