- The Mean, Expected Value, Is (n)(p).
-
- To compute the mean, expected value, find the sum of the products of event and probability of event.
-
- The expected value is the mean. [See a more detailed reason.]

-
- For a binomial distribution, to compute the mean, expected value, multiply the number of trial by the probability of
success on a trial.
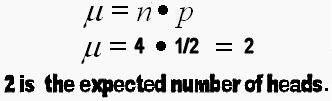
- The expected value is the mean, (n)(p).
Here a fair coin is used so p = 1/2, or .5 and n varies.
Toss 1 coin, n=1 so
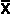 = n(p) = 1(1/2) = 1/2 =.5 = n(p) = 1(1/2) = 1/2 =.5
Toss 2 coins, n=2 so
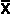 = n(p) = 2(1/2) = 1 = n(p) = 2(1/2) = 1
Toss 3 coins, n=3 so
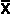 = n(p) = 3(1/2) = 3/2 = 1.5 = n(p) = 3(1/2) = 3/2 = 1.5
Toss 4 coins, n=4 so
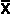 = n(p) = 4(1/2) = 2 = n(p) = 4(1/2) = 2
Toss 5 coins, n=5 so
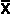 = n(p) = 5(1/2) = 5/2 = 2.5 = n(p) = 5(1/2) = 5/2 = 2.5
|



![[MC,i. Home]](http://www.mathnstuff.com/math/spoken/here/1gif/mcihome.gif)
![[Table]](http://www.mathnstuff.com/math/spoken/here/1gif/table.gif)
![[Words]](http://www.mathnstuff.com/math/spoken/here/1gif/words.gif)

![[this semester's schedule w/links]](http://www.mathnstuff.com/gif/semestr.gif)
![[Good Stuff -- free & valuable resources]](http://www.mathnstuff.com/gif/goods.gif)
![[next]](http://www.mathnstuff.com/math/spoken/here/1gif/next.gif)
![[last]](http://www.mathnstuff.com/math/spoken/here/1gif/last.gif)