Linear         f(x) = mx + b,         (y - y₁) = m(x - x₁)

Starts with a specific amount:     b

Grows the same amount each time (x):     m

Used for cost, revenue, and profit functions

Used to write the equation for a tangent to a curve

   

Exponential         P(x) = P₀e^kx

Starts with a specific amount:     P₀

Continuously grows an amount that varies over time

Approximate e

Growth, Decay, Loans, Savings

 

Discrete         P(t) = P₀(1 + r/n)^nt

Compounding - Growing Discretely

 

    CLICK ON AN IMAGE TO SEE AN ENLARGEMENT.

    In each colored computation box, enter the required info, press/click the gray button, see the resultant computation. Enter negatives as "-x" rather than "- x" on this page. Do NOT write input x values as fractions. Write fractions as good decimal approximations.

    Sometimes questions are given. If possible, answer the question then, with the mouse, swipe the page between the two stars to see the answer to the question.

    To find the "area under a curve," from the point (a,0), draw a perpendicular to the point (a, f(a)) on the curve. One may need to draw it "up" to the curve or "down" to the curve. Repeat this procedure for b. Shade the area between x=a and x=b which is between the curve and the x-axis. This is the area needed. If instead the area between two curves (say f(x) and g(x)), from a to b, is needed, draw the perpendiculars x=a and x=b. Shade the area between the curves.

    For additional information see links at the bottom of this page.

    Enjoy.

    Use the notes and problems and calculators of Linear Functions, on this page, if you need a review or help. A definition of tangent is provided and an example is shown below.

	1. On the circle (x - 3)2 + (y + 4)2 = 4, shown on the left, a tangent has been drawn at about point (4, -5.7). The tangent has a slope of about .58. Write the equation of the tangent line, in point-slope form, at that point. * (y + 5.7) = .58(x - 4)*

	2, For this question you will need to do research at The Square Root Function, y = x, is x1/2 . Write the equation of the tangent line, in point-slope form, at that point when x is 4. Use the calculator on that page to do your research. * at (4,2) m=1/4 so, (y - 4) = .25(x -2).*

symbols:	f(x) = P0bkx
base:	b, b > 0, b 1
y-intercept:	P(0), f(0), the function value when x is 0
nominal rate of change:	k, k 0 if k > 0 there is growth, if k < 0 there is decay
opposite, additive inverse:	f(x) = - P0bkx
multiplicative inverse, reciprocal:	f(x) = P0b- kx
slope:	m = P0kln(b)bkx, b > 0, k 0
domain:	all reals
range:	f(x) > 0
CONTINUOUS or DISCRETE:	continuous
asymptote(s):	horizontal: y = 0
inverse function:	f(x) = P0b-kx
THE exponential function:	f(x) = ex
other resources:	Exponential or Power Functions and Parent Functions, Their Slope Functions, and Area Functions

Function, P(x) = P0bkx             Slope Function, m = P0kln(b)bkx, for each     b >0, b 1, k 0 x =     P0 =     b =     k =

    1. The equation P(x) = 10(2)^4x is an exponential model.
        a. Does it represent exponential growth or decay?*growth*
        b. Write the equation ofthe tangent line, with constants rounded to two places, when x is -.5. * (y - 2.5)= 6.93(x +.5)**

    2. The equation P(x) = 100(3)^-2x is an exponential model.
        a. Does it represent exponential growth or decay?*decay*
        b. Write the equation ofthe tangent line, with constants rounded to two places, when x is 2. * (y - 1.23)= -2.72(x - 2)**

    Until now there has been no emphasis on the difference between discrete and continuous. In the formula P₀(1 + r/n)^nt, some variables are discrete and some are continuous variables. Their classification depends on their use. It is discussed here because some people find it diffcult to choose which formula to choose -- P₀b^kx or P₀(1 + r/n)^nt. Perhaps this is because they chose to memorize the formulas rather than understand them.

    In short, continuous, as in P₀b^kx, means all numbers are used. Discrete means only certain numbers are used.

    P₀b^kx is used for continuous growth. Trees, fish, populations of bacteria or humans grow all the time, unless some external influence exists. A classic example of this is the way rabbit populations continue to multiply (continuously) unless the fox population begins eating them and prohibits their continuous growth. This is described by a logistic model, but that is another story.

    P₀(1 + r/n)^nt is used for discrete growth. Money left in a bank account to grow larger is an example. Interest is paid every once and a while. The more times a year interest is compounded, the more money is earned, up to a point. This is true even though the interest rate each period is smaller. Semiannualy (n=2, twice a year), monthly (n=12, 12 times a year), quarterly (n=4, every 3 months, every quarter) are often used payment intervals. Notice, not every number is used. One can't be paid 1.5 times a year. Use the spread sheet exp.xls to compute or solve all sorts of stuff. Even cash loans on most credit cards only chage interest on a daily (n=365) basis. I think most credit cards charge interest on purchase charge interest on a monthly basis.

    Rate, r, is continuous. It is the percent of the principal that is paid each time interest is paid, each period.

    1. In 2000, P(x) = 7,000(1 + 1.5/100)^x, was first used to describe the population growth, in years, of Keyport, NJ.
a) What would the population be in 2020 if the growth continues at the same rate.
*9427.985045850375 -- In 2020 the population would be about 9428 people. P(t) = 7000(1 + .015/1)^1*20*
b) Experiment. Use this model to predict about when the population of Keyport will first reach 10,000.
*About 24 years, in 2024 the population would be about 10,007 people.*

    2. The number e is the limit, as n goes to infinity, of (1 + 1/n)ⁿ -- as n gets infinitely large.

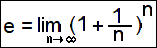
    It is approximately 2.718281828459045...
a)What values of P₀, r, and t must one use in P(t) = P₀(1 + r/n)^nt to make it the equation look like (1 + 1/n)ⁿ? *P₀, r, and t ust each be 1.*
b) How large must n be to obtain 1 decimal digit of e?
*when n is 75, the expression equals 2.700378656300911*
c) How many decimal digits of e does the number 10,000 yield?
*If n is 10,000, e is about 2.7181459268249255, so 3 decimal digits.*

    3. Half-life means the length of time for half of the substance to decay/be changed. Radioactive materials decay. Each has its own half-life. For example, Nobelium has a really short half-life of 23 seconds.
    See source at: Physics Application: Radioactive Decay.
a. Find how much ¹⁴Carbon, carbon 14, remains after 100 years if 400 mg exists at time 0, and it DECAYS, at a rate of -.00012097 per year (base e). *about 395.190 mg.*
b. Find the half-life of ¹⁴Carbon. *
P(100) = about 395.190 mg.
P(1,000) = about 354.42 mg.
P(10,000) = about 199.31 mg, too much.
P(5,730) = about 200.00 mg. The half-life is about 5,730 years.*

    4. ²²⁶Ra has a half-life of 1,620 years and becomes ²²²Ra upon decay. Algebraically find its rate of decay. See source at: Isotopes of radium. *k is about -.00043. * See work.

    5. Which is the better interest? Rate A is 5% nominal interest compounded monthly or Rate B which is 4% nominal interest compounded monthly? Why is it better?
*Rate A is better. It has an APR of 5.09 %. Rate B has an APR of 4.074 %.*

    Hope you learned something and had some fun.

    Don't forget about:

Function and Relation Library.

Trig Functions Library

Exponential or Power Functions

A Bit about e

Lines

Parent Functions, Their Slope Functions, and Area Functions