Exploring Functions ... Presentation Notes

Exploring Functions ...
Lecture Hand Out

Exploring Functions through the Use of Manipulatives: © '94, '95, '97, '98, '00, '04, '12 Agnes Azzolino (asquared@mathnstuff.com)
1) PRETEST Answer these now,: mentally if you wish.; 1. Sketch the graphs of y = 0 and x = 0.; 2. Write the equation for this function.; 3. What effect will the k have in the function y = f(x) + k.; 4. For what numbers is the square of the number greater than on more than the number?; 5. Sketch y = x · sin(x)
HOMEWORK: 6. Before graphing y = x·e^x, predict what the graph should look like. Mentally rehearse an explanation, addressed to another student, which explains or justifies the graph of the function.
2) The Pretest focuses on the content of this session.: It's what the session is about. Please:; 1. Complete the pretest, mentally or in some other manner.; 2. Introduce yourselves to each other and find a partner; 3. Discuss the pretest with your partner.; [Discuss this with your partner (DTWYP).]
3) The materials you will be using today must be returned.: As you leave, please return your materials. If you leave early, please return your materials.; At the end of the session, you will receive a copy of the text of most of the transparencies and a large coordinate plane.
4) Please:: a) Leave the printed coordinate plane in the pocket.; b) Examine and handle the other things in the plastic pocket.; c) Consider how they might be used in mathematics classes.; d) Discuss this with your partner (DTWYP).
5) Consider each question and DTWYP.: What functions have been included?; identity, reciprocal, opposite, absolute value, square,; dilations of the absolute value; What things have not been included but might be?; square root, exponential-log, sine-cosine, half-plane, reciprocal,; half-circles, tangent- cotangent, secant-cosecant; What kinds of things might one do with these manipulatives?
Identify (through point-plotting), Graph, Sketch, Find the inverse function, Estimate the slope, Describe, Discuss, Write an expression, Solve, Predict the graph of, Explain behavior, Show functions with your hands and body.
6) Graph by placement on the coordinate plane. DTWYP.: a. the identity function; b. the opposite function; c. the reciprocal function; d.; e.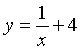; f.; g.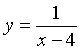; h.; i. y = (x - 4)²; j. y = - (x - 2)² + 3; k. x = y²; m.; n. the distance between a number and 3
7) Sketch each of the above on paper to debrief and DTWYP. Debriefing can not be emphasized enough. Do not leave students "thinking in the concrete." (www.mathnstuff.com/papers/langu/page9.htm) Force a summary in the more abstract written activity of sketching and writing notes, and discussing. (www.mathnstuff.com/papers/langu/page6.htm)
Use of the Slopemeter: Be crude with your slope-generated curve.; [Repeat the slope: Don't use a ruler. Think m=3/4 is also -3/(-4), 6/8.]
8) Solve the statements by graphing 2 functions on the same plane:: [a.] |x - 3| = 4; [b.] |x - 3| = 0; [c.] |x - 3| = -4; [d.] |x - 3| = |x + 2|; [e.] |x - 3| = ( x + 2 )² + 1
9) Use the identity function to compare, describe, then discuss.: A) Graph the identify function and a specific function; on the same coordinate plane,; B) Compare these two functions.; C) Use these thoughts to describe the function. DTWYP.
10) We are not through for the day but we have finished with the manipulatives. Please place your materials back in the pockets. Defrief by listing some ideas we considered. DTWYP as the manipulatives are collected.
11) Before we continue, I'd like to answer any quick Questions.
12) Math "Exercises"(www.mathnstuff.com/papers/langu/exerc.htm): a) Use your body, arms, and hands to represent the graph of:; 1. y = 0; 2. x = 0; 3. y = x; 4. y = -x; 5. y = x²; 6. x = y²; 7. y = -2x²; 8. y = - |x|; 9. y = 2|x|; 10. y = |x| - 2; 11. y = |x| + 2; 12. y = 1/x; b) Please be seated.
13) Dilation by a nonconstant function is wonderful.: Use the composition of functions to sketch an unknown function.; a) What does a cubic look like and why? DTWYP.; y= x³ = x(x²); b) What does a rational function look like?; y = (x +3)(x-4)/(x-2); c) Why does the tangent look that way?; Tangent to the unit circle,; y = tan x = (sin x)/(cos x); d) What are envelope functions?; How do they look?; y = e^x(sin x); Consider the graphs of; sin(x), 4sin(x), x · sin(x),; x² · sin(x), ln(x) · sin(x),; e^x · sin(x)
Other toys:: Sine Law- Ambiguous Case & Sketchpad trig stuff,; Polynomial & Rational Function through Dilation; Spreadsheets for Dialations Creating Polynomials & Rationals
14) Words to the Wise and Wrap Up. Words to the Wise Manipulatives must be available in order to be used. Expect breakage and loss of material. Plan free play before work. Start with short activities and build on these. Always close with an abstraction activity. Self-evaluate after each lesson.
15) We must teach our students to:: Play; Experiment; Suppose; Create; Sketch; Draw; Write Notes; Write Sentences; Debrief; Dream; Think and; Visualize
You've sampled the power of the manipulative and seen it used as it should be in conjunction with introspection, communication, and technology. I hope you learned or relearned a little math, might consider using a strategy demonstrated here today, and had a little fun. Thank you for coming.