pdf of this page   video of this page

 

What's A Fraction?

A fraction is a number. It is a number written as the ratio or comparison of two numbers. The top number is compared to the bottom number. The numerator is compared to the denominator.

 

What's the Job of the Top Number?

The number on the top of the fraction is the numerator, the NUMBERER . It states "how many pieces are involved."

 

What's the Job of the Bottom Number?

The number on the bottom of the fraction is the denominator, the NAMER . It states "the name of the size of the piece." It is the number of equal pieces in one whole."

 

So, What Is A Fraction?

A fraction is a number written so as to compare the number of pieces involved to the number of pieces in one whole.

The fraction one-half, written in symbols as 1/2, means "one piece, where it takes two pieces to make a whole." The fraction a half, written in symbols as 1/2, means "one piece, where it takes two pieces to make a whole."

The fraction one-fourth, written in symbols as 1/4, means "one piece, where it takes four pieces to make a whole." The fraction one-quarter, written in symbols as 1/4, means "one piece, where it takes 4 pieces to make a whole."

The fraction three-quarters, written in symbols as 3/4, means "three pieces, where it takes four pieces to make a whole."

The fraction six-eighths, written in symbols as 6/8, means "six pieces, where it takes eight pieces to make a whole."

 

When Are Two Different Fractions Equal?

Two fractions are equal when they name the same number. It is often the case that two fractions are equal. One-half (1/2) names the same number as two-quarters (2/4), or as three sixths (3/6), or as four-eights (4/8). Three-fourths (3/4) is equal to six-eights (6/8) because they are different ways of expressing the same number.

 

Does It Really Matter Which Way You Write The Fraction?

Yes! Examine the two examples below.   It must be written "number of pieces / number of pieces in one whole."

 

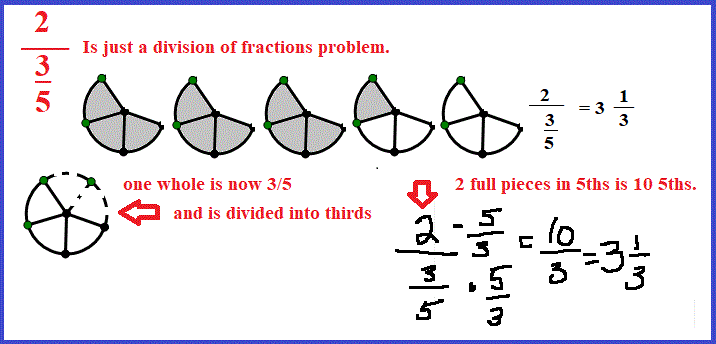
What Does Mean If You Have a Fraction In the Fraction?

It is called a complex fraction and is still "number of pieces / number of pieces in one whole."

For example, below, the denominator, number of pieces in one whole, is now a fraction. So one whole is really 3/5.

 

What Does Mean If You Have a Zero in a Fraction

It will be one of these three kinds of expressions and these depend on where the zero(s) are.

• 0/c, where c is a non-zero constant, equals 0.

  

  * It is zero pieces and any non-zero number in one whole.

  * For example, 0/5 = 0, 0/2 = 0 -- 0 pieces and some number of pieces in one whole.

  * 0/c is found in everyday computation. It is just plain zero.

• c/0, where c is a non-zero constant, is UNDEFINED.

  * "You can't divide by 0." There is no computation rule, as one might find for fractions, for division by zero. Division by zero is NOT DEFINED.

  * c/0 is probably first found in middle school when learning order of operations and getting ready for computation in which c/0 has a useful meaning.

  * That happens in computing the slope of a vertical line

           

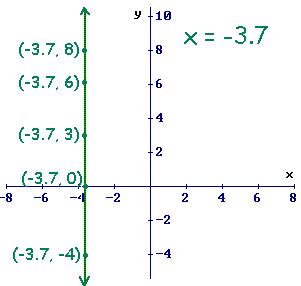
  * c/0 is the reciprocal 0, of 0/c. One might examine 2/0 in a few different ways.

      A. using the function 2/x in the graph below;

      B. in limit.gsp for taking a limit;

      C. using the picture below and the definition of fraction as discussed above, "number of pieces" /"number of pieces in one whole"

      D. using the calculator on this web page. Try a few numbers. Don't forget to try 0 to see what this html math function says.

     

  Enter negatives as "-x" rather than "- x"

• 0/0 is INDETERMINANT.

  * A number of expressions are called INDETERMINANT, meaning the value of the number can not be DETERMINED as is.

  * The expressions are: 0/0, 0^x, ∞ , ∞ /∞ , - ∞ , ∞ ⁰, 0⁰, 1^∞

  * A student probably first sees the fraction 0/0 in calc I when studying limits.

  * Here are some examples of Special Limits. In each case f(0) is 0/0, INDETERMINANT.

  * For more on indeterminant forms see:
        https://calcworkshop.com/limits/limits-indeterminate-forms/ and
        https://byjus.com/maths/indeterminate-forms/#definition.

This is an edited version of a page from the dictionary MATH SPOKEN HERE!, published in 1995 by MATHEMATICAL CONCEPTS, inc., ISBN: 0-9623593-5-1.

© 2005, 2022, Agnes Azzolino www.mathnstuff.com/math/spoken/here/3essay/eso.htm