
The Visual / Auditory / Symbolic / Kinesthetic Approach to Algebra
|

|
|
Term Tiles are NOT the best manipulative for solving systems (a set of equations solved together)! Use Graphing with Manipulatives and/or graphing calculators instead or in addition to Term Tiles. See "Options" for the best method. Linear systems involve many ideas. These include a solution with 2 values, multiple or no solutions, and multiple methods of solution. For a more complete review see "Use Linear Combination to Solve Systems of Equations and Inequalities," "Solve A Systems of Linear Equations by Substitution," and pages linked to them. In addition to the inability to easily find or represent solutions to all systems, solving systems with Term Tiles requires additional tiles. The y / -y tile must be used.  Though Term Tiles are limited in solving linear systems, they are still valuable. Summarized below are the methods presented. The alternate version of linear combination is ideal for solutions which are fractions!
Term Tiles has the advantage of extensive use with "zeroing out" to simplify. Creating a pair of opposites, -1 and 1, or -x and x, or -5x and 5x, and simplifying that pair as 0 is often done with Term Tiles and is quite visible. It is not as visible with pencil and paper computation. The reader is reminded that it is the solver's or the test author's job to choose the method by which the system, set of equations, is to be solved.
The reader is also reminded that manipulatives are made to be outgrown. Term Tiles should be secondary to mental and pencil and paper math. This is particularly true with multiplying none, one, or both equations by constant(s) in linear combination. Before solving an equation, do some warmup work.
 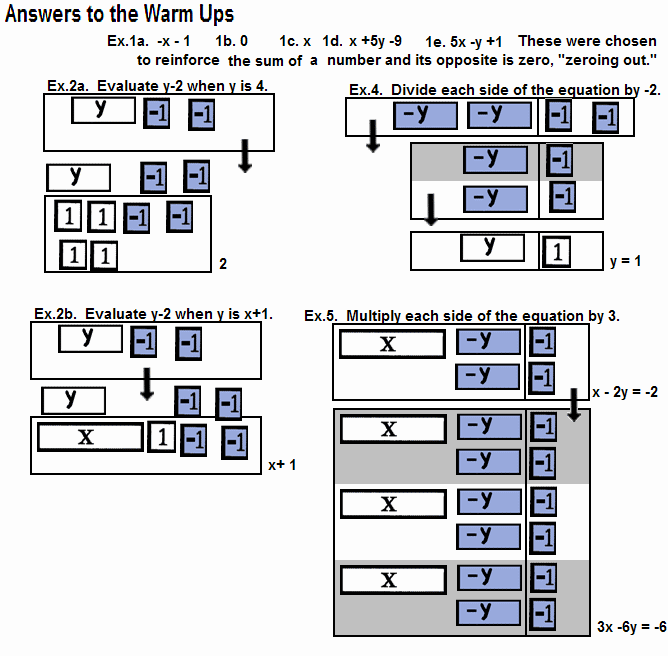 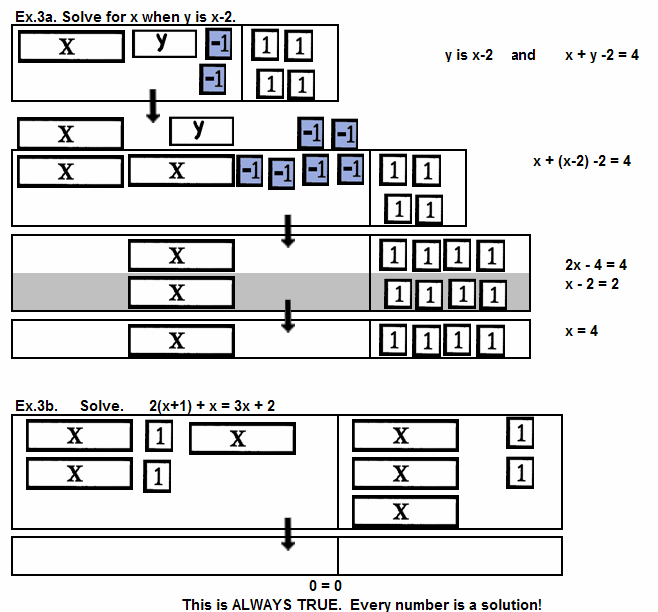 |
 
 www.termtiles.com, Unit 37 © 2008, A. Azzolino |