
The Visual / Auditory / Symbolic / Kinesthetic Approach to Algebra
In algebra, the distributive property is required for the multiplication of even a monomial by a binomial. In arithmetic, the distributive property is used but not required. To set the stage for OPERATIONS (addition, multiplication, division, factoring, etc.) work with REPESENTATION (represent, write, show) is very important. If you have not worked with representing and writing expressions, please do so before continuing with multiplication. Example 1, below, illustrates how the distributive property develops naturally from work with representing and writing algebraic expressions. Consider how representing and writing an expression for "double the sum of a number and three" evolves into "multiply 2 times x+3."
MULTIPLICATION
Multiply. 

Example 3 is even more complicated. It illustrates that distributing a binomial over another binomial is what is usually called multiplying two binomials. 
This author teaches FOIL, prefers FLOI for its use in multiplication of complex numbers, but, emphasizes most that as long as 4 multiplications are completed, it doesn’t matter what order the multiplications are performed. Example 4 illustrates that removing zeros might be required. 
Example 5 "looks funny" and is NOT USUALLY DISCUSSED MANIPULATIVELY. It emphasizes the fact that the displayed product is really the sum of the areas no matter what shape that area takes. 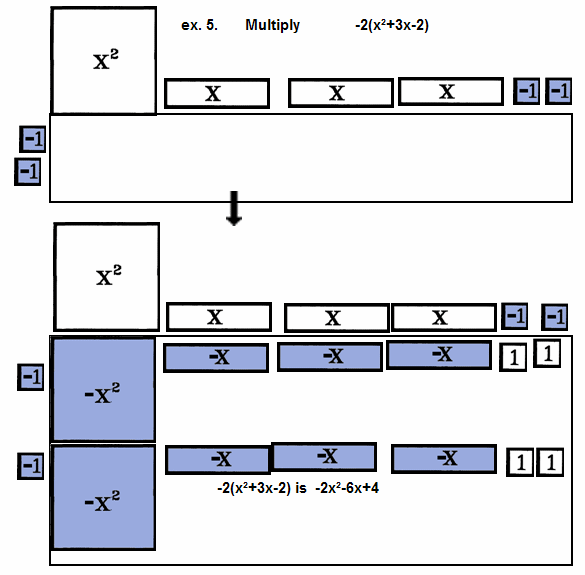
Using the cube token, products not normally considered with manipulatives may be examined. Read "Special Products". 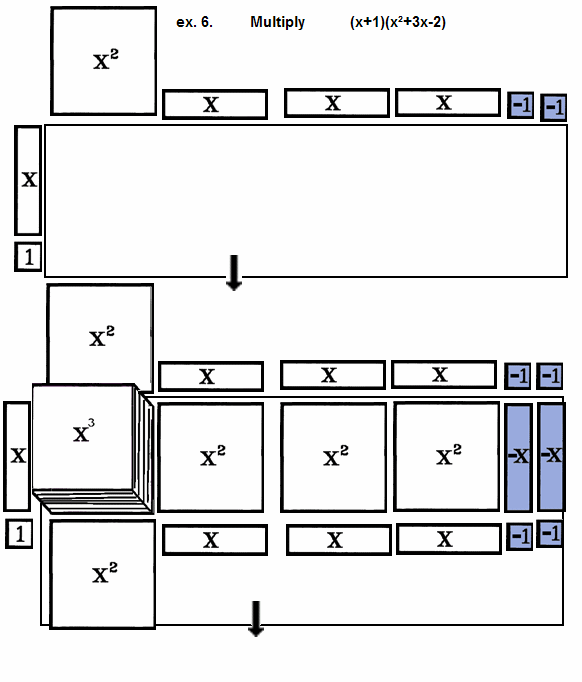 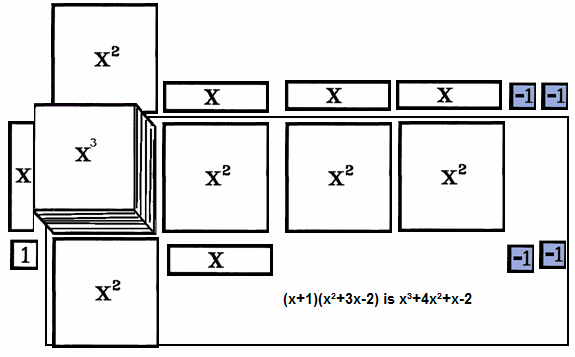
| ||||||||||||||||||||
 
 www.termtiles.com, Unit 17 © 2008, A. Azzolino |

