In arithmetic, variables are not used. In algebra, variables (numbers whose values change) and constants (numbers whose values do not change) are each used.

      In English, the word man might mean a specific man, or it might mean the representative individual, as in "Man created numbers."

      In math, the specific number is a constant, the representaive number is a variable. In math, the phase "a number" means a variable number - all numbers, a number whose value changes sometimes even within a problem.

      Below some algebraic ideas involving numbers are represented manipulatively, in symbols, and in written and spoken words.

the idea	manipulatively	in symbols	in words
one, two, negative three (some constant numbers)		1, 2, -3	one, two, negative three
a number, the opposite of a number		x, 1x -x, -1x	a number, "eks" the opposite of a number, "negative eks"
a number squared or the square of a number the opposite of a number squared or the opposite of the square of a number		x², 1x² -x², -1x²	a number squared or the square of a number or "eks squared" "the opposite of eks squared" or "negative eks squared"
a number cubed or the cube of a number the opposite of a number cubed or the opposite of the cube of a number		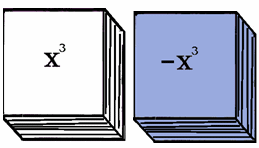x³, 1x³ -x³, -1x³	a number cubed or the cube of a number or "eks cubed" the opposite of a number cubed or the opposite of the cube of a number or "negative eks cubed"
a number to the 4th power the opposite of a number raised to the 4th power		x4, 1x4 -x4, -1x4	a number to the 4th or "eks to the 4th" the opposite of a number to the 4th or "negative eks to the 4th"
two more than a number		x+2 or 2+x	two more than a number "eks plus two" or "two plus eks"
three less than the sum of the opposite of the square of a number and the product of four and a number		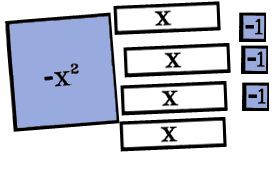-x²+4x-3 or 4x -x²-3or -3 -x²+4x or (-x²+4x) -3	"negative eks squared plus four eks minus three"

      Manipulatively, the orientation or tilt of the tile is not important (except with fractions). In written algebra, the position of a symbol very much matters.

      Here as in written algebra, tiles are generally placed in descending order from left to right. In neither case is it required. What is required manipulatively and with written and spoken algebra is the order dictated by the meaning. More on that later.

      There is more than one way to say something algebraically and verbally. Sometimes more than one statement will be presented, sometimes not.

      Manipulatively there are many ways to say "zero." Here are just a few.

      Usually to represent zero, a pair of matching negative and positive tiles are place one on top of the other, slightly to the side, so the value of each is seen.

      Integer computation and representation is usually introduced and mastered before beginning algebra.

      To do this one must know how to take the opposite.

      With this background in creating zeros and taking the opposite, integer computation can be completed.

      The topic of opposite is extended and clarified under the section entitled "Of…"

      In the units which follow, paired opposites are used to simplify, to factor, and to solve.

      To simplify, find a pair of opposites and "take the zero out" or "zero out" to simplify the expression.

      To factor, add a pair of opposites and "put in a zero" or "zero in" to factor the expression.

      To solve an equation, add the opposite, then "zero out" to simplify the equation.