




| Factored Form | Product | This is called a |
| (x + y)2 | ____________ | ________________________ |
| (x - y)2 | ____________ | ________________________ |
| (x - y)(x2 + xy + y2) | _________________ | _________________________ |
| (x + y)(x2 - xy + y2) | _________________ | _________________________ |
| _________________ | x2 - y2 | __________________________ |
| (x - yi)(x + yi) | x2 + y2 | _________________________ |
| _________________ | x3 + 3x2y + 3xy2 + y3 | _________________________ |
| _________________ | x3 - 3x2y + 3xy2 - y3 | _________________________ |
| _________________ | x4 + 4x3y + 6x2y2 + 4xy3 + y4 | _________________________ |
| _________________ | x4 - 4x3y + 6x2y2 - 4xy3 + y4 | _________________________ |

 may
be simplified but the radical expression
may
be simplified but the radical expression 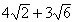 can not be written in a simpler form.
Why not?
can not be written in a simpler form.
Why not? 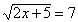 to the method of solving
to the method of solving 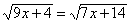 .
. 




 |
 |
 |
|
|
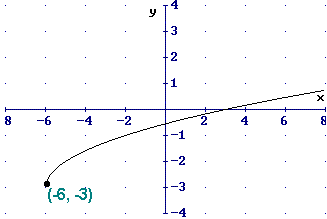
Demonstrate your mastery of the linear, quadratic, absolute value, square root and other functions (including half circles) and things (half-planes) studied in Algebra II, their graphs, and their symbolic expressions. Do this by creating an original and mathematically correct picture and set of expressions (with restrictions if needed). The picture must use graphs of functions as its component parts and must represent something recognizable to the instructor. The set of expressions, with domain and/or range restrictions, must, when graphed, produce the picture. It is suggested that once you have completed your design and generating expressions, you have another person review and correct your work. Here is an example worth 2 or 3 of a 4 possilbe points. The graph paper below may be CLICKED then PRINTED on a separate sheet of paper if desired. One may also RIGHT CLICK on it with the mouse then SAVE the picture as a file. |

|