Ernest Ranucci's Tetra-tetra Flexagon

Once Upon A Time, in the 1970s at an NCTM meeting, I sat in a room with a hundred other math teachers and folded my first tetra-tetra flexagon.   Ernest Ranucci guided us through the paper-folding process and each of us (I think) experienced the joy of making something so beautiful and simple.

The wonder of that moment and the cut and folded flexagon came home with me. The directions for its assembly did not.   I experimented for quite some time before my new flexagon did what I wanted it to do.   So, I take blame for the graphics and the directions but not for the idea.

Materials

• 1 piece of "Scotch" tape about 6 cm long
• scissors
• ruler
• a piece of paper (possibly illustrated on the front and back with the pictures provided)

Making a Tetra-tetra Flexagon

Step 0: Each flexagon requires one piece of tape in addition to the paper flexagon. This tape should be handy for use near the end of the assembly process. It might be placed on the edge of the desk. It requires many steps in assembly. If working with students, after each step, have students place the paper flat on the table. This permits a teacher to scan their work and gets it out of their hands!

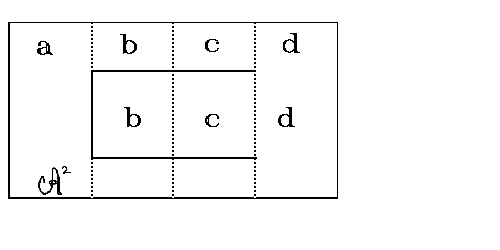
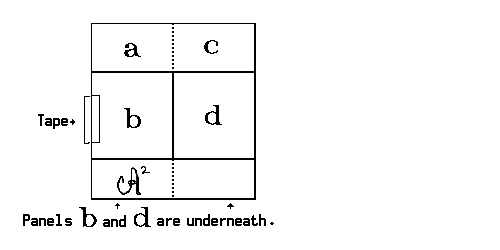
Step 1: Accuracy counts. Fold four panels. Fold a sheet of paper in half then in quarters so the creases are parallel as shown in the figure below. Refold the opposite way so each crease bends forward and backward easily. The better the folding, the more flexible the flexagon.

Step 2: A rectangular window-with-hinge must be cut in the paper. [This window is visible in the figure at step 4.] The side of the rectangle between panels C and D remains intact and acts as a hinge. The side between panels A and B is cut as are the two sides parallel to the top and bottom edges of the paper which stretch from the edge of panel A to the edge of panel D.

In this flexagon the distance from the top of the paper to the rectangular window is 7 cm. The distance from the bottom of the paper to the rectangle is 5.5 cm.

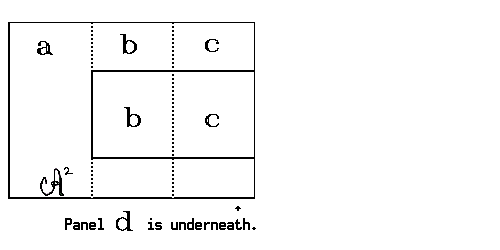
Step 3: Fold the right-most panel under the one next to it. Fold panel D under panel C.

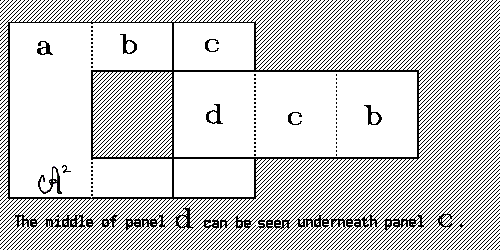
Step 4: Open the window and lay the flexagon flat on the table.

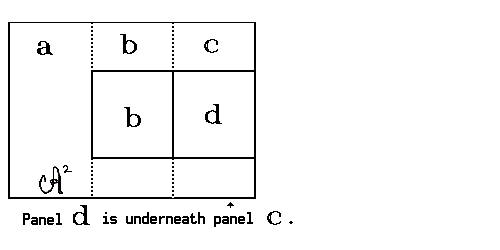
Step 5: Fold the window panels under the rest of the paper. This leaves all of panel A visible, the tops and bottoms of panels B and C visible, and the middles of panels B and D visible.

Step 6: Pull panel B through the window and let it extend at an angle perpendicular to the rest of the flexagon and the desk upon which it rests. It looks like an open window.

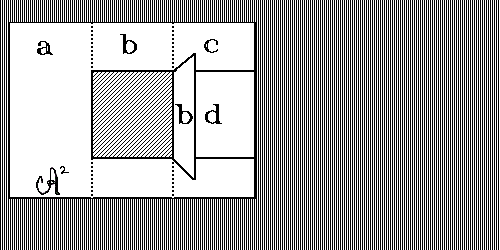
Step 7: Fold panel A to the right. Panel A should be covering the top and bottom of panel B and closing the window through which the middle of panel B was pulled.

Step 8:

1st: Fold panel B flat against the desk on to of panel A.

2nd: Take the tape and hang it over the center of the left edge of panel B so that it is half on and half off panel B.

3rd: Bend the tape around the edge of panel B taping panel B to panel A beneath it.

How to Flex a Tetratetra Flexagon

"Bend the book back on its binding."

1st: Hold the panels as one would hold an open book: hold the right and left edges with the right and left hands.

2nd: Work the creases so the "book" opens and closes easily.

3rd: Bend the "book" back pushing the binding up (breaking the binding). If the flexagon does not separate at the crease between the adjacent panels, assist the separation with your thumbs. If this is still not possible, turn the flexagon over so the back of the panels is visible rather than the front, and try bending it back again.   Either the back or the front will flex to reveal new panels.

These Graphics

Before using the flexagon illustrated in the following pages, it is important to know the answers to the questions it poses. The house drawn above the set containing the numbers 3, 5, and 8 is a "Home on the Range." The next number in the sequence 1, 4, 9, ..., is the next square, 49. The next letter in the sequence, 0, T, T, F, F, S, S, E, ... is N, for nine (one, two , three, etc.).

The figure in the box represents "Stars and Stripes Forever." The empty rectangles are drawn so the user may draw his/her own figures or questions or riddles. Math daffinitions are a lovely idea also. For example, the figures below might be considered "scrambled eggs" and "rectangles [wrecked angles]."

The blank spaces on the flexagon's panels are left so one can draw or write their own commutative or noncommutative figures or words.   Words like "cake" and "pan" noncommutatively switch to "pan cake."

Panel A has: "Questions:"

Panel B has: "Answers:," clouds, flowers, the logos.

Panel C has: arrow, "subtraction," baby fish.

Panel D has: "Don't argue" and "exponents."

The back of panel A has: clouds and lawn mower.

The back of panel B has: empty box, etc.

The back of panel C has: "home on the range," etc.

The back of panel D has: arrow, empty boxes, momma/monster fish.

Printed Tetra-tetra Flexagon

The two gif images printed below should be center and duplicated on the front and back of an 8 1/2" by 11" sheet of paper. The image size is 8" by 10".

The user is reminded that the author/artist gives permission for the material and graphics to be duplicated as is for nonprofit purposes.

For larger, more useful copies of these graphics, place your mouse on the graphic, right click, save the picture as a file with a name of your choice.

Click on image to see just image.